1. Basic Music Notation: Pitch
   1. The Clefs and Their Pitches
   2. The Twelve-Tone Chromatic Scale
   3. Sharps, Flats, Naturals, Key Signatures, and Accidentals
2. Harmony 101: Basic Scales, Chords, & Modes
   1. Basic Scales: Diatonic Major, Natural Minor, & Modes
   2. Harmonic Ratios, or, Pythagoras with a looking glass
   3. Harmonic Intervals & Basic Triads
   4. Dissonance Is Not Bad
   5. Why the Diatonic Scale Became Paramount
   6. Rules for Key Signatures
   7. Augmented Chords & Whole-Tone Scales

Basic Music Notation: Pitch

The Clefs and Their Pitches

I wrote on my page about rhythm that a note’s height signifies its pitch; the type of note signifies its duration. I was oversimplifying slightly, though: to the left of the musical staff, you’ll see a symbol called a clef. By far the most common of these are the treble clef (𝄞) and the bass clef (𝄢).

The clef you see affects what pitch values are written on the staff. In the treble clef, from bottom to top, the five lines represent the notes E, G, B, D, and F, for which Every Good Boy Deserves Favor is commonly used as a menomnic. The spaces in between the lines are F, A, C, and E, which serve as their own mnemonic.

The bass clef’s notes are, from bottom to top, G, B, D, F, and A, for which the similar mnemonic Good Boys Do Fine Always is used. The spaces are A, C, E, and G, for which the mnemonic All Cows Eat Grass is often used.

The clef is usually found at the left of a musical score, like so:

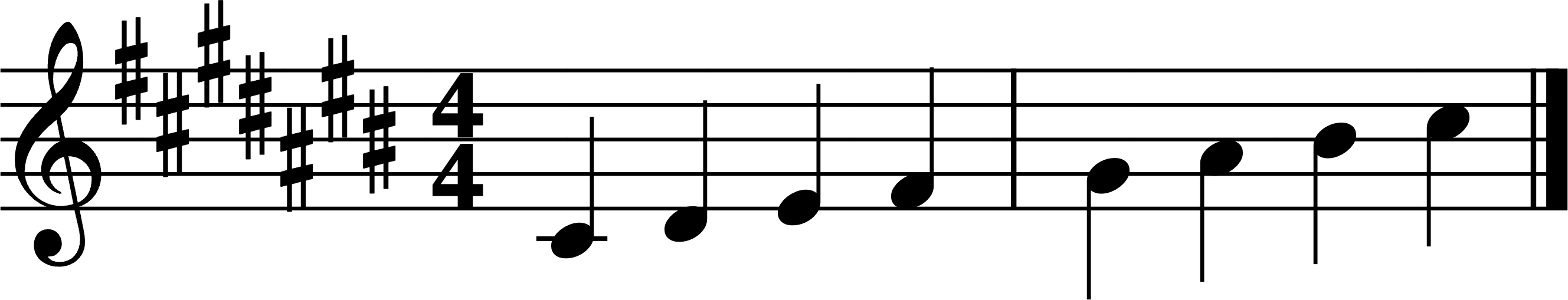
This is the C♯ major scale, starting on C♯4, the note immediately above middle C. But we’re getting ahead of ourselves. We need to start with an explanation of pitch.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

The Twelve-Tone Chromatic Scale

The vast majority of modern Western music uses twelve-tone equal temperament (12-TET). Scientifically, this means every octave is subdivided into twelve equal ratios – more specifically, for those of you mathematics nerds out there, each note’s pitch is exactly 2¹⁄₁₂ (≈1.05946309436) that of the note a half-step below it. If that doesn’t make sense to you, don’t worry; you needn’t understand advanced mathematics to play or write music, though I’ll use some simpler mathematics to explain harmony and dissonance later.

Although the octave is divided into twelve notes (which, in total, sum up to the chromatic scale), most Western music uses a scale that selects only seven of those notes for the majority of its melodies and harmony. The white notes on the piano correspond to the C major scale (or, alternately, the A natural minor scale), which each correspond to an arrangement of those seven notes. These notes are highlighted on the table below.

This immediately raises at least three questions:

1. Why are the distances between highlighted notes uneven? (Observant readers may have already noticed that B and C and E and F are closer to each other than the other highlighted notes are.)
2. Why do most of the notes have two names?
3. What do ♯ and ♭ mean?

I’ll answer these in the reverse order.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Sharps, Flats, Naturals, Key Signatures, and Accidentals

♯, the sharp symbol, raises a note a half-step above its normal value (e.g., A → A♯). ♭, the flat symbol, lowers it a half-step below its normal value (e.g., A → A♭). ♮, the natural symbol, negates either of these symbols and sets a note back to its non-sharp, non-flat value.

If any of the above symbols appears immediately to the left of a note, it’s considered an accidental and lasts until the end of a measure. There are different rules if they are set in the key signature, ordinarily seen immediately to the right of the clef. The key signature applies to all notes of a given pitch (thus, in a key signature specifying an F♯, all Fs become F♯s unless otherwise specified). Generally, naturals aren’t specified in a key signature, but sometimes the key signature changes mid-song (which is called modulation). In such cases, naturals may be specified (although they don’t have to be). Key signature modulation is almost always preceded by a double bar (𝄁), after which the new key signature is declared.

The key signature provides a useful sort of shorthand for musicians. If we had to specify F♯ every measure, not only would it be repetitive, but once an F♮ finally occurred, it’d be a musical Boy Who Cried Wolf of sorts – our brains would have gotten so used to the F♯ that they’d just fill it in. Key signatures and accidentals notate a piece’s most commonly used pitches more logically and concisely while making its outliers stand out more.

This, in turn, is part of why most of the notes have two names: raising C by half a step and lowering D by half a step are two ways to refer to the same pitch, but they’re semantically different: the key signature of the passage affects which one is more logical to use (it wouldn’t make sense to use both F♯ and G♭ in the same measure). D, G, and A are the only notes with a single name because they’re the only ones sandwiched between two black piano keys. Here are the notes again; this time, white piano keys have lighter backgrounds.

And here’s a third presentation, with only one name for each white key.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Harmony 101: Basic Scales, Chords, & Modes

Basic Scales: Diatonic Major, Natural Minor, & Modes

Again, the highlighted keys correspond precisely to the C major scale. Scales using the same ratios as C major are referred to as diatonic major scales, meaning they feature the following intervals in the following order:

Each scale, in turn, has modes, or variants starting from different notes of the scale. The diatonic major scale is also called Ionian mode; the other ubiquitous mode is Aeolian mode, or the natural minor scale, which starts at the major scale’s sixth degree (A, in the case of C major) and runs up an octave to the next A:

I’ve conducted a detailed analysis of the diatonic major scale’s modes, but it’s quite technical and may be partly incomprehensible without advanced music theory knowledge. In brief, modes of scales (besides the first) are results of starting the scale on a different note. For instance, Aeolian (natural minor), the diatonic major scale’s sixth mode, starts on its sixth note rather than its first. The diatonic major scale’s seven modes crop up by far more often than any other scale’s; since roughly the mid-sixteenth century, they’ve been called:

(Although many of these names date back to Ancient Greece, the ancient Greek names don’t line up with the modern ones, for reasons that are sufficiently off-topic here that I’d advise interested parties to read “A Crash Course in Ancient Greek Harmony” from my aforementioned analysis on modes.)

Here are examples using the C major scale:

The word chromatic is derived from the Ancient Greek word χρωματικός (romanized: khrōmatikós), meaning either relating to colour or relating to one of the three types of tetrachord in Greek music. Bearing this in mind, it occurs to me that some readers may find it helpful to see the chromatic scale’s twelve degrees represented with twelve evenly spaced Oklab hues: C, C♯/D♭, D, D♯/E♭, E, F, F♯/G♭, G, G♯/A♭, A, A♯/B♭, B.

Notes are often followed by numbers representing the octave they belong to. C4 is defined as middle C. Since the diatonic major scale is considered the center of Western harmony, and the piano’s white keys in turn receive foremost importance, the note below C4 is not B4 but B3. (This should qualify as a violation of the Geneva convention against OCD sufferers.) The note above C4, meanwhile, is C♯4. Thus, the entire chromatic scale goes C4, C♯4, D4, D♯4, E4, F4, F♯4, G4, G♯4, A4, A♯4, B4, C5.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Harmonic Ratios, or, Pythagoras with a looking glass

To return to the seemingly arbitrary note spacing of the C major scale (and thus, the white keys on the piano), it reflects deeply considered geometric ratios that date back to the Pythagoreans, two and a half millennia ago.

It may be helpful to understand, first, that the octave (from Latin octavus, or eighth; so named because it’s the distance between the diatonic major scale’s first and eighth notes) is based on the fundamental ratio of 2:1. That is, Western music defines A4’s pitch as 440 Hz. A3, found an octave below A4, is 220 Hz, or 440 / 2. A5, found an octave above A4, is 880 Hz, or 440 * 2. Several other foundational intervals approximate other superparticular (i.e., n+1:n) ratios; these were first specified by the Pythagoreans approximately 25 centuries ago.

In the following table, “WS” stands for how many whole steps the top note is above the bottom note, “P” stands for “Pythagorean” (i.e., the “ideal” ratios), “M” for “Modern” (i.e., the twelve-tone equal temperament employed by modern tuning), “R” for “Ratio”, and “Q” for “Quotient”. Mathematics nerds may find it interesting that our modern twelve-tone equal temperament is within about 0.0015 of the Pythagorean ratios for perfect fourths and fifths, while its ratios for the major and minor third are off by approximately 0.01.

In short, the ear finds the superparticular ratios between small integers consonant, or harmonically pleasing. However, drifting too far from these fundamental ratios (or direct multiples thereof) creates dissonance, or pitch combinations that most listeners find unsettling. The tritone, a √2:1 ratio, is so dissonant that historically, it was the interval most often called diabolus in mūsicā (Latin: the devil in music), although contrary to popular belief, it was never banned, nor could composers be excommunicated just for using it. Jazz bassist and music theorist Adam Neely has called the minor ninth, a 2^{¹³⁄₁₂}:1 (≈2.11892618872) ratio, the most dissonant interval.

Also, note that large intervals’ names become increasingly disconnected from their whole step counts (as seen above) because two contiguous pairs of white keys on the piano (B to C and E to F) are a half-step apart, and the intervals are (with the obvious exception of the tritone) named for their degrees on the C major scale: e.g., since F is its fourth degree, C to F is a perfect fourth. Thus, a minor sixth contains four whole steps.

Further explanation of the standard interval nomenclature: “Perfect” intervals are the same in both Aeolian and Ionian mode; a minor nth represents the interval from Aeolian mode’s first to nth scale degrees, while a major nth represents the interval from Ionian mode’s first to nth scale degrees. The tritone does not occur within either mode from above its first note, so it is usually not measured as a fourth or a fifth (although it is sometimes called an augmented fourth or a diminished fifth); in fact, it only occurs above the first note in Lydian and Locrian (and as a different degree in each: it’s Lydian’s fourth note and Locrian’s fifth).

For those curious about the approximate pitches of various notes, I’ve got you covered.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Harmonic Intervals & Basic Triads

However, understanding harmony and dissonance doesn’t require fully understanding the mathematics behind them. It can help, but the important point is this: the ear finds octaves, perfect fifths, perfect fourths, and thirds pleasing, while intervals that stray too much from these in specific ways feel unsettling. Let’s examine several triads, or chords comprised of three notes, for some examples:

• A diminished chord (e.g., B3, D4, and F4) by itself contains two minor thirds (B3 to D4, D4 to F4), but it also contains a tritone (B3 to F4), which strays from both the perfect fourth and perfect fifth by enough to make the entire chord feel dissonant overall.
• A major chord (e.g., C4, E4, and G4) is built of a major third below a minor third. Thus, we have a major third (C4 to E4), a perfect fifth (C4 to G4), and a minor third (E4 to G4). Since all of these intervals are harmonically pleasing, so is the chord itself.
• A minor chord (e.g., A3, C4, and E4) is built of a minor third below a major third. Thus, we have a minor third (A3 to C4), a perfect fifth (A3 to E4), and a major third (C4 to E4). Thus, it feels harmonically pleasing for the same reason as the major chord, but for reasons too complex to cover here, the major chord sounds “happier”.
• A major chord’s first inversion (e.g., E4, G4, and C5) happens when we move its base form’s bottom note up an octave. Thus, we have a minor third (E4 to G4), a minor sixth (E4 to C5), and a perfect fourth (G4 to C5). All of these are harmonically pleasing intervals.
• A minor chord’s first inversion (e.g., C4, E4, and A4) contains a major third (C4 to E4), a major sixth (C4 to A4), and a perfect fourth (E4 to A4). These are likewise all harmonically pleasing.
• A major chord’s second inversion (e.g., G4, C5, and E5) happens when we move its base form’s top note down an octave. Thus, we have a perfect fourth (G4 to C5), a major sixth (G4 to E5), and a major third (C5 to E5). Again, all harmonically pleasing.
• A minor chord’s second inversion (e.g., E4, A4, and C5) contains a perfect fourth (E4 to A4), a minor sixth (E4 to C5), and a perfect fourth (A4 to C5). Yet again, harmonically pleasing.

• A suspended second (sus2) chord (e.g., C4, D4, and G4) consists of a major second (e.g., C4 to D4), a perfect fifth (e.g., C4 to G4), and a perfect fourth (e.g., D4 to G4). The first of these intervals is somewhat dissonant, but the other two are consonant enough to make the chord harmonic overall.

  However, this chord also carries with it an expectation of a resolution. Since it does not clearly establish tonality (in this case, whether we’re in a major or a minor key), it is usually followed by either its respective major or minor chord.

• A suspended fourth (sus4) chord (e.g., C4, F4, and G4) consists of a perfect fourth (e.g., C4 to F4), a perfect fifth (e.g., C4 to G4), and a major second (e.g., F4 to G4). Everything said above about the suspended second chord also applies to the suspended fourth.

This list omits an important three-note chord type: the augmented chord. Augmented chords do not appear in the diatonic major scale or any of its modes, so I’ll address them below. Let’s first return to the B3-D4-F4 diminished chord above. If we throw a G3 below those notes, we wind up with G3-B3-D4-F4, a G7 chord. From G3, we have a major third to B3, a perfect fifth to D4, and a minor seventh to F4; from B3, we have a minor third to D4 and a tritone to F4; and from D4, we have another minor third to F4. This chord feels far less dissonant than the diminished chord, but it also carries an expectation of a resolution: the ear expects C major to follow it.

If our key is C major, then C major itself is the scale’s tonic or root chord. The fifth chord of the scale, G major, is called the dominant chord. The dominant to tonic progression is the single most common chord progression in music, and it’s the easiest way to establish the home key, both as a composer and as an active listener. When the chord progression jumps down by a perfect fifth (or up by a perfect fourth), there’s a good chance you’ve found your root.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Dissonance Is Not Bad

I want to be extremely clear here, though: although I’ve spent a long time explaining what creates harmony, dissonance is not inherently bad. In fact, in many cases, it can be what you want. Two particularly famous examples of this include John Williams’ theme for Jaws and Bernard Herrmann’s theme for Psycho. Another great example is Krzysztof Penderecki’s Threnody to the Victims of Hiroshima, which uses droning dissonance to incredibly unsettling effect. The point shouldn’t be to avoid dissonance entirely; it’s to avoid it where it’s unwanted.

(“Krzysztof Penderecki” is pronounced roughly “Kshishtoff Pendetetski”, but native English speakers can be forgiven for pronouncing his first name “Krishtoff”, since English never places “ksh” together within a single syllable – the closest you’ll find is something like “Berkshire”, which uses them in separate syllables. If all else fails, just don’t rhyme his last name with “Becky” or pronounce the z’s as z’s and you won’t embarrass yourself too badly.)

Additionally, there’s a difference between blue notes (i.e., acceptable dissonance) and sour notes (i.e., fingernails on a chalkboard), but until I’ve delved into non-diatonic scales, any explanation I could provide would boil down to (with all apologies to Justice Potter Stewart) “I know it when I hear it”. In some cases, sour notes may even be what you want – there are no universal rules in music, apart from “there are no universal rules in music, apart from ‘there are no universal rules in music, apart from…’”. The point isn’t to avoid any particular kind of interval entirely: it’s to know how the interval makes listeners feel, so you can employ it to its greatest effect.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Rules for Key Signatures

Ordinarily, key signatures must follow certain rules. It is not permitted to mix flats and sharps; moreover, notes must be flatted or sharped in a specific order, following what’s referred to as the circle of fifths.

This table provides several demonstrations of the circle of fifths. G is a perfect fifth above C; it’s also the key above C in this table in both the major and minor columns. Continue traveling upwards by fifths from G and you’ll find D, which is the next row in the table. Up from that we have A. And so on.

This applies in the accidentals column as well. B♭ is a perfect fifth above E♭, which in turn is a perfect fifth above A♭, and so on. The same applies for the sharps, but in reverse order. And another corollary applies here: wherever I’ve written “a perfect fifth above”, we can replace it with “a perfect fourth below”.

At this point, I hear some readers asking themselves, “Wait, is all harmony centered around the circle of fifths?” I’m tempted to just answer “yes” and drop the mic, but that’d be a slightly glib oversimplification. Nonetheless, the circle of fifths is a foundational component of musical harmony, and you will start to see it everywhere. In fact, I’d say that after the notes of the treble and bass clefs, the circle of fifths order F-C-G-D-A-E-B (and its reverse, B-E-A-D-G-C-F) is the single most important note order to memorize in music. It occurs everywhere.

Note that the first five sharps have the same pitch values as the first five flats, just in reverse order. Meanwhile, the last two sharps and flats are alternate names for white keys on the piano keyboard. This is not coincidental: there are only five black keys per octave. This also means that any key signature with six accidentals must rename one of the white keys on the keyboard. As much as I dislike this, it keeps pieces in F♯/G♭ major (or D♯/E♭ minor) from needing to use a rash of accidentals, so it’s still less terrible than the alternative.

Why the Diatonic Scale Became Paramount

The inevitable question arises as to why the diatonic scale became so special that the piano keys were literally fashioned around the C major scale. The ultimate answer is that it’s been a central scale since the Pythagoreans’ day, and it’s an especially stable one because, as it turns out, it’s not actually possible to have a more even arrangement of seven notes within our twelve-tone equal temperament. As it turns out, there are numerous advantages to this, but my explanation will be much more comprehensible if I explain the basics of Roman numeral chord shorthand first:

• Chords are numbered for the scale degree corresponding to their root notes
• An uppercase Roman numeral generally signifies a major chord, e.g.:
  • I is a major chord rooted on the scale’s first note
  • IV is a major chord rooted on its fourth note
• Lowercase Roman numerals generally signify minor chords, e.g.:
  • ii is a minor chord rooted on the scale’s second note
  • vi is a minor chord rooted on its sixth note
  Some people choose to write all chords as uppercase Roman numerals. This obfuscates their tonality for anyone who doesn’t know what mode the piece is written in (or doesn’t remember any of the modes besides major and natural minor). Please don’t be one of those people.

So, now that I’ve got the basics out of the way, the diatonic major scale is 12-TET’s smallest scale to contain chords meeting all six of the following criteria:

1. three major chords separated by perfect fifths:
   1. the I chord (rooted on the first note)
   2. the IV chord (rooted on the fourth note), a perfect fourth above (or a perfect fifth below) the I chord
   3. the V chord (rooted on the fifth note), a perfect fifth above (or a perfect fourth below) the I chord
2. three minor chords also separated by perfect fifths:
   1. the vi chord (Aeolian i), a major sixth above (minor third below) its first note (Aeolian’s third note)
   2. the ii chord (Aeolian iv), a perfect fourth above (perfect fifth below) Aeolian’s i chord
   3. the iii chord (Aeolian v), a perfect fifth above (perfect fourth below) Aeolian’s i chord

The inevitable response is, “That sounds pretty cool, but how important is it really?” The answer, as it turns out, is, “These are very likely the most important traits Western harmony’s most widely used scale could possibly possess.” In brief, the chords rooted on a scale’s base note and those rooted a perfect fourth and a perfect fifth above it are by far its most important chords for establishing tonality. In fact, Western music’s single most common chord progression is almost certainly IV-V-I and variants thereof (IV-V7-I, iv-v-i, iv-V-i, etc.).

Of course, that just raises further questions, among them:

• Why are the chords rooted on the diatonic major scale’s second, third, and sixth notes so important?

  Aeolian mode (natural minor) starts on its sixth note, and Aeolian’s fourth and fifth notes are its second and third notes. Thus, a ii-iii-vi progression in major is a iv-v-i progression in Aeolian mode. This is called the relative minor – same key signature, different base key. This is contrasted with the parallel minor, which keeps the same base key but requires a change of key signature (three more flats, three fewer sharps, some combination thereof, or, very occasionally, something more convoluted – e.g., since G♭ major’s parallel minor is a monstrosity involving two double-flats, most composers would instead switch to F♯ minor).

• What’s so funny about peace, love, and understanding special about twelve-tone equal temperament?

  It possesses numerous advantages, as it turns out: it enables us to play close aproximations of all five of the Pythagoreans’ consonant intervals from all twelve notes of the chromatic scale, and their ratios don’t vary based on the root note. Twelve-tone equal temperament also closely approximates the Pythagorean scale’s remaining intervals, 9:8 (a major second) and 256:243 (a minor second; this seemingly random ratio consists of the leftover interval within a perfect fourth and a perfect fifth after the appropriate number of major seconds has been accounted for). Moreover, because the notes are equally spaced, these intervals’ tonal qualities will not vary based on their roots – that is, a perfect fourth expresses the same ratio whether its root note is C or F♯.

(When I write a page on chord progressions, I’ll explain all of this in much greater depth.)

A few additional notes on chord shorthand:

• Diminished chords are written as lowercase Roman numerals followed by a superscript letter o, e.g., vii^o, rooted on the scale’s seventh note
• A superscript “7” after a Roman numeral signifies the addition of a minor seventh above the chord’s root note. (A superscript “maj7” or “∆7” signifies the addition of a major seventh.)
• A superscript “6” after a Roman numeral signifies that the chord is in first inversion. (Rarely, a subscript “3” may also be added.) A superscript “6” and subscript “4” signify that it is in second inversion. (By extension, a superscript “5” and subscript “3” may signify that it is in its standard form, but this almost never happens.) Alternately, the chord may be followed by “b” to signify that it is in first inversion or “c” to signify that it is in second inversion.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

The Circle of Fifths: Down the Rabbit Hole

Let’s return to the above table on key signatures and expand it. The following table is adapated from my page on modes. In order, its column headers stand for “Lydian”, “Ionian” (Major), “Mixolydian”, “Dorian”, “Aeolian” (Minor), “Phrygian”, “Locrian”, and “Key Signature”. (The gray “Dorian” column is a pun on a classic Oscar Wilde novel.) Don’t panic about its information density: not all of it will yet be meaningful to you. However, you may note that I presented the modes in a different order from the one seen above, and also that following them from left to right results in another passage up the circle of fiths. If you’re wondering whether these facts are related, the answer is an unequivocal “yes”.

Readers may notice a few additional points about this table:

1. The central row showing a key signature with no sharps or flats lists the keys in the same order that I’ve shown the accidentals in.
2. The “Locrian” column equates to the last sharp added to a key signature, and the “Lydian” column equates to the last flat added to a key signature. (I’ve shown flats in reverse order here for brevity’s sake.)
3. In the first seven columns, the key listed below a particular table cell is always the same one listed to its left (if both exist), and the key listed above it is always the same one listed to its left (if both exist).
4. Although several note pairs (B♯/C♮, C♯/D♭, D♯/E♭, E♮/F♭, E♯/F♮, F♯/G♭, G♯/A♭, A♯/B♭, and C♭/B♮) represent a single pitch, they’re nonetheless semantically different in music notation, not just due to key signature differences, but also because they represent different scale degrees (e.g., F♯ is C Lydian’s fourth scale degree, while G♭ is C Locrian’s fifth scale degree, even though both notes are six half-steps above the root).
5. The first unsharped, unflatted note in the circle of fifths (F) and the last unsharped, unflatted note (B) are a tritone apart from each other. They’re also the only notes on the piano where either:
   1. traveling up by three white keys does not equate to traveling up by a perfect fourth, and traveling down by four white keys does not equate to traveling down by a perfect fifth (F), or
   2. traveling down by three white keys does not equate to traveling down by a perfect fourth, and traveling up by four white keys does not equate to traveling up by a perfect fifth (B).
   Furthermore, in the row with zero accidentals, F is the first mode (Lydian) and B is the last (Locrian).

If you’re beginning to suspect that absolutely none of this is coincidental, you’re absolutely right. Furthermore, if you’re beginning to wonder if this chart could be extended above B♯ or below F♭… it could, but many musicians (myself included) don’t like to talk about it. Double-sharp (𝄪) and double-flat (𝄫) symbols exist, but I personally feel their only place in music notation is for pieces that don’t conform to the diatonic scale, so that’s the only place I’ll acknowledge their existence. But this feels like the ideal time to cover non-diatonic scales.

Back to top · My discography · Marathon soundtracks · Contact me · Website index

Augmented Chords & Whole-Tone Scales

I avoided mentioning one of the four fundamental types of chord above, because it cannot appear in the diatonic major scale or any of its modes. An augmented chord (e.g., C, E, and G♯) contains two major thirds (C to E, E to G♯) and a minor sixth (C to G♯). It doesn’t feel dissonant, but it does feel eerie. It belongs to one of the whole-tone scales, which consist of six evenly spaced scale degrees (either C, D, E, F♯, G♯, A♯, or C♯, D♯, F, G, A, B). Whole-tone scales can establish a dreamy or playful mood; because all scale degrees are evenly spaced, they also have no clear root key, which creates a sense of being suspended in space or time.

(To be continued.)

Further reading: Musical Modes and the Circle of Fifths

Back to top · My discography · Marathon soundtracks · Contact me · Website index